By the end of the lesson, the learner should be able:
- to identify integers in different situations;
- to represent integers on a number line.
By the end of the lesson, the learner should be able:
Consider moving forward as a positive direction whereas moving backward as a negative direction.
Positive whole numbers, negative whole numbers and zero are called integers.
Consider the following integers:
–5, –1, +4, –3, 0, +1, +3, +2, +5, –4, –2
Use greater than (>) and less than (<) signs to compare the following integers:
Solution
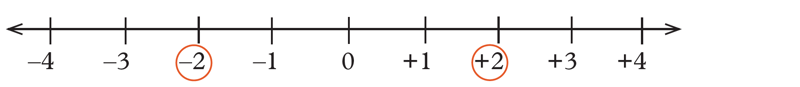
(–2) is on the left of (+2)
(–2) is less than (+2)

(–7) is to the left of (–1)
(–7) is less than (–1)
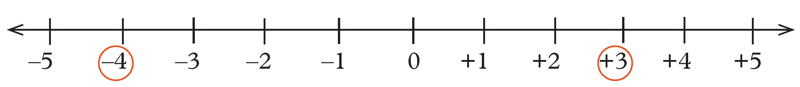
(+3) is on the right of (–4)
(+3) is greater than (–4)
Use a number line in the exercise.

Choose the files you want to add. Supported formats are txt, html, htm, pdf, odt, odp, ods, xls, xlsx, ppt, pptx, pps, doc, docx, rtf, png, jpg, jpeg and gif.
| Name | |
|---|---|
| remove |
NB! Links must begin with: “http://”!
Opiq uses essential cookies to make our website work, to help keep you safe, to analyse user interaction and to improve user experience.
Cookie is a small file which is sent from users computer to the website server. It includes necessary information for the website to operate and includes information about the user and their preferences.
Most of the cookies are necessary for the operation of Opiq. It is possible to deny analytical cookies and in that case your usage data is not used to develop and improve Opiq services. Read more